EXTREMES OF MULTIDIMENSIONAL STATIONARY GAUSSIAN
RANDOM FIELDS
Abstract: Let 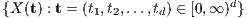 be a centered stationary Gaussian field
with almost surely continuous sample paths, unit variance and correlation function
be a centered stationary Gaussian field
with almost surely continuous sample paths, unit variance and correlation function 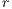 satisfying
satisfying  for every
for every 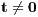 and
and 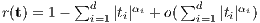 , as
, as
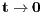 , with some
, with some ![α1,α2,...,αd ∈ (0,2]](files/38.1/HTML/38.1.10.abs6x.png) . The main result of this contribution is
the description of the asymptotic behaviour of
. The main result of this contribution is
the description of the asymptotic behaviour of 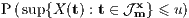 ,
as
,
as 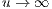 , for some Jordan-measurable sets
, for some Jordan-measurable sets  of volume proportional to
of volume proportional to
![d -1
P(sup(X (t) : t ∈ [0,1] ) > u) (1+ o(1))](files/38.1/HTML/38.1.10.abs10x.png) .
.
2010 AMS Mathematics Subject Classification: Primary: 60G15; Secondary: 60G70,
60G60.
Keywords and phrases: Gaussian random field, supremum, limit theorem, asymptotics,
Berman condition, strong dependence.