UNIVERSITY
OF WROCŁAW
 
Main Page
Contents
Online First
General Information
Instructions for authors


VOLUMES
43.2 43.1 42.2 42.1 41.2 41.1 40.2
40.1 39.2 39.1 38.2 38.1 37.2 37.1
36.2 36.1 35.2 35.1 34.2 34.1 33.2
33.1 32.2 32.1 31.2 31.1 30.2 30.1
29.2 29.1 28.2 28.1 27.2 27.1 26.2
26.1 25.2 25.1 24.2 24.1 23.2 23.1
22.2 22.1 21.2 21.1 20.2 20.1 19.2
19.1 18.2 18.1 17.2 17.1 16.2 16.1
15 14.2 14.1 13.2 13.1 12.2 12.1
11.2 11.1 10.2 10.1 9.2 9.1 8
7.2 7.1 6.2 6.1 5.2 5.1 4.2
4.1 3.2 3.1 2.2 2.1 1.2 1.1
 
 
WROCŁAW UNIVERSITY
OF SCIENCE AND
TECHNOLOGY

Contents of PMS, Vol. 23, Fasc. 2,
pages 257 - 368
 

LARGE DEVIATION THEOREMS FOR WEIGHTED COMPOUND POISSON SUMS

Olivier Bonin

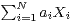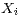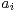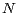Abstract: In this paper we present two large deviation results for weighted compound sums sum N a X i=1 i i , where X i ’s are i.i.d. (possibly lattice) random variables, a i ’s are non-negative real numbers, and N is a Poisson variable. These results are generalizations of approximations for non-weighted compound sums and for non-compound weighted sums.

2000 AMS Mathematics Subject Classification: 60F10, 60F05, 60G50.

Key words and phrases: Large deviation expansions, compound process, weighted sums, saddlepoint method.

Download:    Abstract    Full text   Abstract + References