UNIVERSITY
OF WROCŁAW
 
Main Page
Contents
Online First
General Information
Instructions for authors


VOLUMES
43.2 43.1 42.2 42.1 41.2 41.1 40.2
40.1 39.2 39.1 38.2 38.1 37.2 37.1
36.2 36.1 35.2 35.1 34.2 34.1 33.2
33.1 32.2 32.1 31.2 31.1 30.2 30.1
29.2 29.1 28.2 28.1 27.2 27.1 26.2
26.1 25.2 25.1 24.2 24.1 23.2 23.1
22.2 22.1 21.2 21.1 20.2 20.1 19.2
19.1 18.2 18.1 17.2 17.1 16.2 16.1
15 14.2 14.1 13.2 13.1 12.2 12.1
11.2 11.1 10.2 10.1 9.2 9.1 8
7.2 7.1 6.2 6.1 5.2 5.1 4.2
4.1 3.2 3.1 2.2 2.1 1.2 1.1
 
 
WROCŁAW UNIVERSITY
OF SCIENCE AND
TECHNOLOGY

Contents of PMS, Vol. 26, Fasc. 1,
pages 97 - 112
 

CURSE OF DIMENSIONALITY IN APPROXIMATION OF RANDOM FIELDS

M. A. Lifshits
E. V. Tulyakova

Abstract: Consider a random field of tensor product-type X(t), t (- [0,1]d, given by

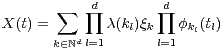
sum prod d prod d X(t) = c(kl)qk fkl(tl) k (- Ndl=1 l=1
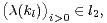
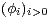
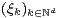
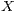
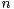
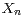
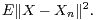
where ( ) c(kl)i>0 (- l2, (fi)i>0 is an orthonormal system in L2[0,1] and (qk)k (- Nd are non-correlated random variables with zero mean and unit variance. We investigate the quality of approximation (both in the average and in the probabilistic sense) to X by the n -term partial sums Xn minimizing the quadratic error 2 E||X - Xn || . In the first part of the paper we consider the case of fixed dimension d. In the second part, following the suggestion of H. Woźniakowski, we consider the same problem for d-- > oo . 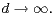We show that, for any fixed level of relative error, approximation complexity increases exponentially and we find the explosion coefficient. We also show that the behavior of the probabilistic and average complexity is essentially the same in the large domain of parameters.

2000 AMS Mathematics Subject Classification: 41A63, 60G1S.

Key words and phrases: Random fields, Gaussian processes, fractional Brownian sheet, linear approximation error, complexity.

Download:    Abstract    Full text   Abstract + References