UNIVERSITY
OF WROCŁAW
 
Main Page
Contents
Online First
General Information
Instructions for authors


VOLUMES
44.1 43.2 43.1 42.2 42.1 41.2 41.1
40.2 40.1 39.2 39.1 38.2 38.1 37.2
37.1 36.2 36.1 35.2 35.1 34.2 34.1
33.2 33.1 32.2 32.1 31.2 31.1 30.2
30.1 29.2 29.1 28.2 28.1 27.2 27.1
26.2 26.1 25.2 25.1 24.2 24.1 23.2
23.1 22.2 22.1 21.2 21.1 20.2 20.1
19.2 19.1 18.2 18.1 17.2 17.1 16.2
16.1 15 14.2 14.1 13.2 13.1 12.2
12.1 11.2 11.1 10.2 10.1 9.2 9.1
8 7.2 7.1 6.2 6.1 5.2 5.1
4.2 4.1 3.2 3.1 2.2 2.1 1.2
1.1
 
 
WROCŁAW UNIVERSITY
OF SCIENCE AND
TECHNOLOGY

Contents of PMS, Vol. 28, Fasc. 2,
pages 305 - 324
 

ASYMPTOTIC PROPERTIES OF PERIODOGRAM FOR ALMOST PERIODICALLY CORRELATED TIME SERIES

Łukasz Lenart

Abstract: The main purpose of this paper is to establish the asymptotic properties of the expectation and variance of periodogram for nonstationary, almost periodically correlated time series. We expand our consideration to the whole bifrequency square (0,2p]2 . We show the exact form of asymptotic covariance between two values of periodogram which are calculated at different points. This result implies that periodogram is not consistent in mean square sense for any point from bifrequency square (0,2p]2 . 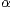Finally, under the moment and a -mixing condition, we prove the consistency of smoothed periodogram.

2000 AMS Mathematics Subject Classification: Primary: 62G20; Secondary: 62G05.

Keywords and phrases: Periodogram, almost periodically correlated time series, consistency.

Download:    Abstract    Full text   Abstract + References