Abstract: Burkschat et al. (2003) have introduced the concept of dual generalized order statistics (dgos) to unify several models that produce descendingly ordered random variables (rv’s) like reversed order statistics, lower 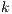-records and lower Pfeifer records. In this paper we derive the limit distribution functions (df’s) of bivariate central and bivariate intermediate 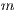-dgos. 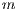It is revealed that the convergence of the marginals of the -dgos implies the convergence of the joint df. Moreover, we derive the conditions under which the asymptotic independence between the two marginals occurs.

2000 AMS Mathematics Subject Classification: Primary: 62G30; Secondary: 62E20.

Keywords and phrases: Dual generalized order statistics, dual generalized central order statistics, dual generalized intermediate order statistics.