UNIVERSITY
OF WROCŁAW
 
Main Page
Contents
Online First
General Information
Instructions for authors


VOLUMES
44.1 43.2 43.1 42.2 42.1 41.2 41.1
40.2 40.1 39.2 39.1 38.2 38.1 37.2
37.1 36.2 36.1 35.2 35.1 34.2 34.1
33.2 33.1 32.2 32.1 31.2 31.1 30.2
30.1 29.2 29.1 28.2 28.1 27.2 27.1
26.2 26.1 25.2 25.1 24.2 24.1 23.2
23.1 22.2 22.1 21.2 21.1 20.2 20.1
19.2 19.1 18.2 18.1 17.2 17.1 16.2
16.1 15 14.2 14.1 13.2 13.1 12.2
12.1 11.2 11.1 10.2 10.1 9.2 9.1
8 7.2 7.1 6.2 6.1 5.2 5.1
4.2 4.1 3.2 3.1 2.2 2.1 1.2
1.1
 
 
WROCŁAW UNIVERSITY
OF SCIENCE AND
TECHNOLOGY

Contents of PMS, Vol. 28, Fasc. 2,
pages 235 - 256
 

OCCUPATION TIME FLUCTUATIONS OF POISSON AND EQUILIBRIUM BRANCHING SYSTEMS IN CRITICAL AND LARGE DIMENSIONS

Piotr Miłoś

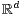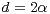Abstract: Limit theorems are presented for the rescaled occupation time fluctuation process of a critical finite variance branching particle system in Rd with symmetric a -stable motion starting off from either a standard Poisson random field or the equilibrium distribution for critical d = 2a and large 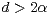d > 2a dimensions. The limit processes are generalised Wiener processes. The obtained convergence is in space-time and finite-dimensional distributions sense. Under the additional assumption on the branching law we obtain functional convergence.

2000 AMS Mathematics Subject Classification: Primary: 60F17, 60G20; Secondary: 60G15.

Keywords and phrases: Functional central limit theorem; occupation time fluctuations; branching particles systems; generalised Wiener process; equilibrium distribution.

Download:    Abstract    Full text   Abstract + References